How To Establish Sample Sizes For Process Validation Using LTPD Sampling

The first article in this series, Risk-Based Approaches To Establishing Sample Sizes For Process Validation (June 2016),  provided and established the relationship between risk and sample size. This article will demonstrate the use of lot tolerance percent defective (LTPD) to establish sample sizes for process validation.

LTPD, rejection quality level (RQL), and unacceptable quality level (UQL) represent the level of defects routinely rejected by a sampling plan. Lots at or above the LTPD are rejected most of the time. For example, LTPD_0.05 will be rejected 95 percent of the time.  LTPD is the opposite of the acceptable quality limit (AQL), which is the level of quality that will be accepted 95 percent of the time. In other words:

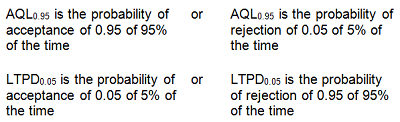
Consumer’s risk is the probability that a defective product will be accepted by the customer. Producer’s risk is the probability that a good product will be rejected by the customer. 

Table 1: AQL and LTPD Protection Comparison Using 95% Confidence

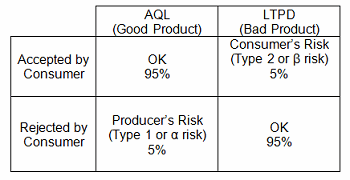
LTPD sampling specifies the minimum sample size to ensure with 95 percent confidence that a lot having a percent defective equal to the LTPD will not be accepted. Note: 95 percent confidence is generally used; however, other confidence levels can and may be appropriately used.

I would like to suggest that variable sampling plans should be utilized whenever possible for two reasons. First, variables data will yield much more information than a comparable sample using attribute data. Secondly, with fewer samples, associated costs of inspection, measurement, and testing can be substantially reduced.

It should also be noted that a simple random sample is meant to be an unbiased representation of a group. A sampling error can occur with a simple random sample if the sample doesn't end up accurately reflecting the population it is supposed to represent. In other words, each piece that is to be inspected, measured, or tested must have the same chance of being selected and representative of the batch or lot.

Start With FMEA

Before we begin, we must establish our definitions of risk and their acceptable quality limit LTPD_0.05, the level of quality that will be rejected 95 percent of the time. These definitions can and should vary based upon organizational needs.  A good place to determine the risk level is from the failure mode and effects analysis (FMEA). FMEA (design, process, user) is a systematic group of activities designed to recognize, document, and evaluate the potential failure of a product or process and its effects.

FMEA uses a risk priority number (RPN), which is composed of frequency, detection, and severity. The higher the RPN, the higher the risk; however, a high severity in conjunction of low probability of occurrence and high probability of detection may still necessitate the appropriate controls for high risk.  Table 2 depicts an example FMEA with the associated risk levels.  Once the risk level has been determined (low, medium, high), the appropriate LTPD_0.05 can be selected using Table 4.  Figure 1 depicts the linkage between FMEA, risk, and LTPD_0.05.

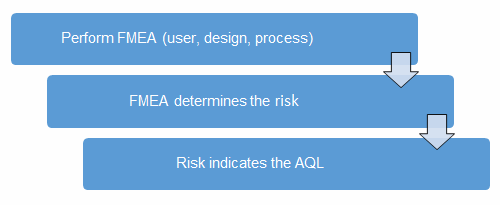
Figure 1: Risk process for determining the appropriate AQL

Table 2: Example FMEA

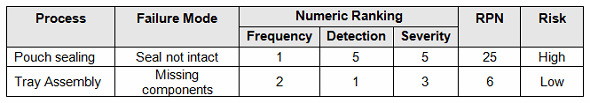
Table 3 shows an example of risk level definitions with accompanying defect classifications. These definitions can and will vary based upon the product(s) produced and their intended and unintended uses.

Table 3: Example of Risk Level Definitions

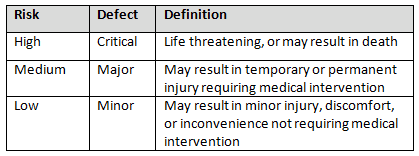
Table 4 depicts an example LTPD_0.05 based upon risk. Different LTPDs can and should be utilized based upon the organization’s risk acceptance determination threshold, industry practice, guidance documents, and regulatory requirements.

Table 4: Example LTPD_0.05 Based Upon Risk Acceptance (95% Confidence)

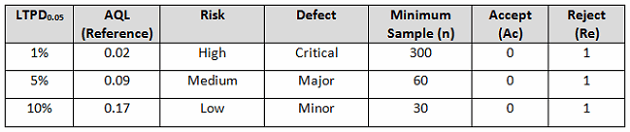
Acceptance sampling is used to assess the quality based on a confidence, quality level, sample size (n), acceptance (Ac) number, and rejection (Re) number. C=0 sampling is based on the premise of accepting the lot if zero defects are found during the inspection, and rejecting the lot if one or more defects are found during the inspection. (Another way to define C=0 is the acceptance (Ac) number (a) is zero (0); in other words, we accept the lot if zero (0) defects are detected.)  LTPD sampling plans provide more protection to the consumer, which is especially important when health and human welfare are involved.

Examples

A tray assembly process is determined to pose a low risk, where a minor defect may result in minor injury, discomfort, or inconvenience not requiring medical intervention. According to Table 4, an LTPD_0.05 of 10% should be used, which requires a minimum of 30 samples. It is expected that a normal production run will consist of 250 pieces. With a lot size of 250 and an LTPD_0.05 of 10 percent, 30 pieces must be randomly selected and inspected with zero (0) defects detected to meet the process validation acceptance criteria. 

It should be noticed that if the lot size were increased to 500 pieces, no additional inspection is required. By strategically determining lot sizes, significant cost savings can be realized due to decreased inspection, measuring, and testing costs.

A pouch sealing process is determined to pose a high risk, where a critical defect may be life threatening or may result in death. According to Table 4, an LTPD_0.05 of 1 percent should be used, which requires a minimum of 300 samples. With an LTPD_0.05 of 1 percent, 300 pieces must be randomly selected and inspected with zero (0) defects detected to meet the process validation acceptance criteria. 

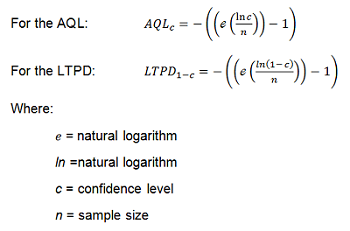
The Math Behind Table 4

So, you may be wondering how the LTPD and AQL were calculated in Table 4. The calculations used are relatively straightforward and can be performed using an inexpensive scientific calculator. 

Choosing The Right Requirements For Your Process

I want to reinforce that different LTPDs can and should be utilized based upon an organization’s risk acceptance determination threshold, industry practice, guidance documents, and regulatory requirements. It should be noticed that the lot size does not affect the minimum sampling requirements.

This article series has introduced several different methods for establishing sample sizes for process validation. Previous articles in the series include:

• Risk-Based Approaches To Establishing Sample Sizes For Process Validation
• How To Establish Sample Sizes For Process Validation Using The Success-Run Theorem
• How To Use Reliability-Based Life Testing Sampling For Process Validation
• How To Establish Sample Sizes For Process Validation Using C=0 Sampling Plans
• How To Establish Sample Sizes For Process Validation Using Statistical Tolerance Intervals
• How To Establish Sample Sizes For Process Validation Using Variable Sampling Plans

References:

1. ANSI/ASQ Z1.4-2008: Sampling Procedures and Tables for Inspection by Attributes
2. Durivage, M.A., 2014, Practical Engineering, Process, and Reliability Statistics, Milwaukee, ASQ Quality Press
3. Durivage, M.A. and Mehta B., 2016, Practical Process Validation, Milwaukee, ASQ Quality Press
4. Durivage, M.A., 2016, Risk-Based Approaches To Establishing Sample Sizes For Process Validation, Life Science Connect

About the Author:

Mark Allen Durivage is the Managing Principal Consultant at Quality Systems Compliance LLC and an author of several quality-related books. He earned a B.A.S in Computer Aided Machining from Siena Heights University and a M.S. in Quality Management from Eastern Michigan University. Durivage is an ASQ Fellow and holds several ASQ certifications including; CQM/OE, CRE, CQE, CQA, CHA, CBA, CPGP, and CSSBB. He also is a Certified Tissue Bank Specialist (CTBS) and holds a Global Regulatory Affairs Certification (RAC). Durivage resides in Lambertville, Michigan. Please feel free to email him at mark.durivage@qscompliance.com with any questions or comments or connect with him on LinkedIn.